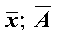
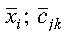
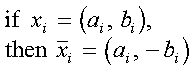
The complex conjugate of the complex matrix element cjk
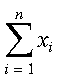
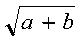
A-1
A-T
The mathematical and programming notations used in this book are consistent
with traditional mathematical and programming usage. These conventions are
explained below, along with special abbreviations that are associated with
specific values.
| Item | Description |
|---|---|
| Greek letters: alpha, sigma, omega, OMEGA | Symbolic scalar values |
| |a| | The absolute value of a |
| a*b | The dot product of a and b |
| xi | The i-th element of vector x |
| cij | The element in matrix C at row i and column j |
| x1 ... xn | Elements from x1 to xn |
| i = 1, n | i is assigned the values 1 to n |
| y <-- x | Vector y is replaced by vector x |
| xy | Vector x times vector y |
| ak | a raised to the k power |
| ex | Exponential function of x |
| AT; xT | The transpose of matrix A; the transpose of vector x |
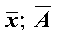 | The complex conjugate of vector x; the complex conjugate of matrix A |
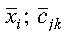 | The complex conjugate of the complex vector element
xi, where:
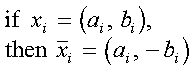 The complex conjugate of the complex matrix element cjk |
| xH; AH | The complex conjugate transpose of vector x; the complex conjugate transpose of matrix A |
| I | Identity matrix |
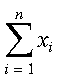 | The sum of elements x1 to xn |
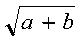 | The square root of a+b |
|
A-1
| The inverse of matrix A |
|
A-T
| The transpose of A inverse |
| |A| | The determinant of matrix A |
| m by n matrix A | Matrix A has m rows and n columns |
| sin a | The sine of a |
| cos b | The cosine of b |
| SIGN (a) | The sign of a; the result is either + or - |
| address {a} | The storage address of a |
| size(a, dim) | The result equals the number of elements in a along a specified dimension dim or if dim is not present the total number of array elements in a. |
| max(x) | The maximum element in vector x |
| min(x) | The minimum element in vector x |
| ceiling(x) | The smallest integer that is greater than or equal to x |
| floor(x) | The largest integer that is not greater than x |
| iceil(m,n) | The smallest integer that is greater than or equal to m/n; that is, iceil(m,n) = ceiling(m/n) |
| int(x), x > 0 | The largest integer that is less than or equal to x |
| m --> (p, i) | m is mapped into (p, i) |
| mod(x, m) | x modulo m; the remainder when x is divided by m |
| infinity | Infinity |
| pi | Pi, 3.14159265 |